lukas – Page 5 – Saul Theory

December 10, 2017September 24, 2021

The Chinese Economic Miracle

Bloomberg recently pointed out that the Chinese economy is “bigger than” the economy of the United States. Today we’re going to explore why. Actually they did a pretty good job with the article; my standards might be low but I thought it was better than I expected from that source. My experience (tl;dr) is that they are certainly right about that conclusion, but hey I will draw it out into a lengthy blog post.

Another Bloomberg article claims that the Chinese economy isn’t and won’t be bigger than the US economy for 10 years. I completely disagree with most all the numbers they presented then (a year and a half ago now). But hey – it’s really hard to come up with accurate numbers in economics – so hard that almost nobody even tries to put error bars on their stats. And unfortunately it’s even harder to draw useful conclusions from them.

Mea Culpa

It seems like these days every 瓜老外 (melon [head] foreigner) has a blog or youtube channel about life in China. But really, what do you expect from us? I’ve been here less than a year, and only speak beginner level Mandarin. You think you are going to arrive at some high level knowledge of east Asian cultural anthropology here? Good, I didn’t think so either. But just maybe, you haven’t even been to China – so in this case the perspective I offer could – perhaps – give you something. It could also be that my relative ignorance on the topic could allow me to explore some perspectives which would be off limit to the seasoned professional. This happens from time to time in technical fields of academia so, why not? Ok on to the show.

The Chinese economy is MUCH larger than the United States’ economy.

Well this is the immediate impression upon seeing the place from the air, and walking around in a few cities. There are way more people, and each one of them does way more commerce. There are so many more restaurants, more pushcarts, more vehicles, more fleets of BMWs and Audis, more well-dressed fruit-stand operators on every corner, more advertisements, and more electronics. More bikes, more motorbikes, more electric vehicles, more exercise watches, more digital payment systems, and more language schools. More dancing light shows on buildings, more buildings, more and better and bigger roads, more and better trains and subways. More shopping malls, more farmers markets, and more fireworks. More all-night bars, more cigarettes, more trash, more plastic, more taxis, and more dancing seniors on the streets. This is not just the mega-cities but even “small” cities, even those which have basically no sign of existence on the English speaking web.

You get the idea? I feel like one of the Spanish explorers arriving in Tenochtitlan (now Mexico city) – if you haven’t read any of these accounts, they were astounded by the magnitude and variety of commerce taking place in what was then the biggest city in the world, or at least much bigger than anything in Europe. I have the same questions as I look around here. How is this possible? What could possibly support all this infrastructure, energy use, food consumption, etc. etc. ? Is this Technological Tower of Babel really going to stay upright? OK so not everything is bigger here. There are fewer police, fewer psychoanalysts, fewer jails, fewer golf courses, and not as much live music. Not as much cannabis. Not as much drug addiction (especially coffee). Not as much begging in the street.

Does an economy have a size?

Lets take a step back. Does it even make sense to represent some complex economy with a scalar number? We love to put scalars on things as then we can compare them directly, but the question of whether or not the comparison has any meaning in the context of your choosing is another one. It’s not clear that putting a number on the activities of people inside an arbitrary border is a useful exercise, and it’s also not clear that one would rather have this number be larger or smaller.

But lets ignore these most important considerations for now and just go on anyway.

GDP

I know right? GDP is somehow an important measure of the economy, everybody knows. Well as much as you might understand this as unspoken in a lot of discourse on economics, in actuality most economists don’t come right out and say it. But hey, let’s take a look. There it is right in figure one of the Bloomberg article.

The first thing we see is that it is measured in dollars. The dollar is a privately issued or fiat currency, and so there is no cost or limitation to issuing more of them. It appears at first glance to a scientist that GDP can’t possibly be a useful figure, if it is measured in such a unit. We can tell this is a broken measurement, for example we see that the GDP of areas undergoing hyperinflation shows massive increase – although there isn’t much change in the underlying economic activity. Zimbabwe’s economy grew by a million percent or so in 2015, right? Is this a useful number?

It turns out that there are a couple assumptions we can make, or definitions if you like, that return GDP to a useful status.

We must assume that the activities of the wealthiest people don’t count (or aren’t what we are interested in) when we are talking about this measure of size of an economy.

If we make this assumption, then GDP becomes meaningful again – and it only breaks as a useful measurement when the money issuing class (if this exists) begins to be a rapidly changing influence on the economy.

OK so this is rather forced I know, but the thing is we want to find some number – even if it’s not clear what it represents at least it is something – that we can try to use as a yardstick. So let’s look at the reported GDP. Note that “reported” has various levels of significance and difference in different parts of the world. Who are we reporting it to? Who is tallying and why should we be honest? To see the kind of money that is floating around China, here’s another article from the same source. But let’s look at reported GDP, just for fun.

China – 75 Trillion 人民币

USA – 0 人民币

Haha you see what I did there? Not many people report earning CNY back stateside do they. OK so let’s try to compare:

USA – 20 Trillion 美元 ($)

For a quick back of the envelope calculation to compare these numbers, we see that a cheap pack of smokes in China goes for 5 to 10 CNY, so we could get 7 to 15 trillion packs of smokes with that kind of money. In USA the cheap smokes go for 10 to 20 dollars so you could get 1 to 2 trillion. This puts the Chinese economy at some 7 times the size of the US economy.

Not fair you say? Well sure, tobacco is heavily taxed back in the land of the free. When we are looking at GDP, why not look at the Big Mac index – another champion of The Economist. This isn’t really fair either because 麦当劳 is more of a fancy foreign restaurant in China. In fact it enjoys such a reputation on the European Continent as well (which you can spot this if you squint at the big mac index or wind up travelling through Europe). They claim it’s 20 kuai for a big mac, which is definitely very high here. A typical restaurant will give you an sizable lunch for about half that. But anyway, let’s compare that to the US at $5.3 (where?) for the sandwich. This would mean you can get about 4 trillion big macs in china for their reported GDP, compared to, well really this is within any error bars – these two numbers are about the same.

Back to the topic!

Sorry about that diversion! OK we’re not going to quantitatively prove the Chinese economic miracle exists. However we are going to try to explain it, thanks to a Russian colleague of mine, who insisted we answer this question one night around a table with a group of Moroccans, Americans, and Russians at a local bar.

Without further ado, here are some potential explanations for the Chinese economic miracle:

One-Party Politics

Some people say that the one-party system enables quick action and has avoided stagnation and helped quick adoption of new technologies. Well, by itself this isn’t going to do much but perhaps with a lot of other things in place it could help. Some might argue this is basically the way things work everywhere to dome degree, as there are factions within the party.

Luck / Timing / Not Invaded by Orcs

Not being invaded by barbarian foreigners can really help the development. Also one can never deny an element of luck and timing. Perhaps the revolution came at the right moment in terms of global economics and technology?

Population

Well this is certainly a dominant factor. However comparison to India here is worthwhile.

Language

Perhaps the requirement of literacy and the symbolic work that it includes helps people become better functioning members of a society. There’s a real chance that the language itself has something to do with the economic miracle. “Culture” is of course the obvious answer to our problem, but really now we are trying to figure out what that means.

Soil

A wise person once said the health of the people is the health of the soil. After all, we are what we eat. Thanks to millennia of floods from the Himalayas running through mainland china, and not as much desertification, there is a lot of deep and mineral rich topsoil here. This is hugely important for feeding folks and stopping mineral deficiencies, and so plays at least some role in the economics.

Leadership

You might ask for example, why they have built so many great trains over here while they haven’t built any in the United States. Why not? Well the obvious answer worth considering, is that the leaders cared in one place while in the other they didn’t. Whether this should be attributed to less corruption, better education, or a better selection process, I will leave to you to figure out.

OK we’ll stop there for now. Let me know what you think, if there’s an economic mircale here at all, or if you have other factors in mind that could have given rise to this alleged miracle.

October 22, 2017December 19, 2017

Bell’s Gambit Declined

Introduction

Hello Everyone.

Some of you may be aware of a mission I have recently joined: to help scientists clean up and add precision to the dialog concerning our chess game of understanding quantum mechanics.

In a recent essay (中文）I outlined a three pronged attack to better teach this topic and better understand the implications. One of these prongs concerns elimination of the terms “nonlocality” and “noncausality” which some authors have been pushing as necessarily implied by experiments and theory of quantum mechanics. The attack we can make with a tempo on this heavily popularized line is a deadly one, and I wrote up and published one version of it under the name “The Emperor Has No Nonlocality” in 2015 (preprint). Today I’m going to go over this game and explain for you how I recommend you play this position on the board.

To briefly review before we get started, “The Emperor Has No Nonlocality” outlines a potentially crushing move against those who wish to push the line that quantum mechanics is incompatible with local realism. We accomplish this by locating the heart of the problem, and demonstrating that it can be explained with local physics.

Well really, I didn’t locate this heart, David Mermin located it for us. Many professors and authors have pointed to Mermin’s immortal description of the EPR paradox as the most accessible one, and the most clear for students or those who might be unfamiliar with some of the notation used by other authors. In this article he even explicitly invites us to solve the puzzle using local physics, which is exactly the path we take, following the work of David Bohm and others. It is my hope that this solution of his gedankenexperiment will enable others to see this opening with a tempo to the heart of nonlocality, and enable us to proceed to tackle more formidable foes and to a much stronger understanding of the physics.

The Gambit Begins

But wait, you say: doesn’t everybody know that quantum mechanics is inconsistent with local physics? What about Bell’s theorem and the associated experimental work?

Indeed, this is where the game gets interesting. Bell’s immortal 1964 paper putting forth his gambit is in my opinion extremely well written. I’ve gone through it dozens of times over more than two decades. It is a miniature, to the point, and doesn’t indulge in the sometimes tempting academic pursuits of lengthening, obfuscation, and over-referencing. If I had to find another paper of this style I would be tempted to mention the Mathematical Theory of Communication by Claude Shannon, and if you know something about my preferences you will know this is the highest compliment I can find for an academic paper. To put it succinctly, Bell plays a sharp game. His game comes in first getting us to accept a set of notations and a definition, after which he leaves us struggling to deal with the consequences.

John Stewart Bell

The gambit appears immediately in Bell’s equation one, in which he presents a “definition of local realism”, in the context of the Stern-Gerlach experiment:

$A = A(\vec a,\vec \lambda )$

$B=B(\vec b,\vec \lambda)$

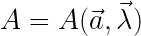
where

$A, B = \pm 1$

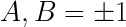
Here we have the results of two measurements $A$ and $B$ , measurements of the deflection of two electrons which have passed through Stern-Gerlach devices and been registered on detectors.

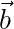These measurements, according to Bell’s gambit, depend only as calculable functions on the orientation of the relevant measurement apparatus ( $\vec a$ or $\vec b$ ) and the internal state of the electron prior to entering the device ( 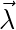$\vec\lambda$ ). 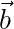The result of this measurement, we are told, can only be up or down (1 or -1), and Bell emphasizes that the measurement $A$ cannot depend on the orientation of the $B$ apparatus ( $\vec b$ ), nor can the measurement 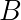$B$ depend on the orientation of the 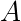$A$ apparatus ( $\vec a$ ).

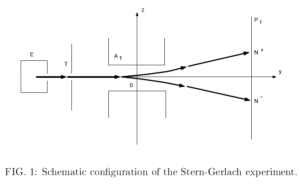
It certainly is consistent with the use of the word “local” in that if these measurements did depend on the other settings far away, there would be nonlocal behavior at work.

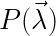Furthermore, Bell provides us with a means to arrive at a probabilistic distribution – by considering a set of many electron states each $\vec \lambda$ , with some distribution $P(\vec\lambda)$ . This he handily provides in his equation 2. At this point he tells us already what fate is in store for us – that the probability arrived at by this formalism CANNOT be that predicted by the formalism of quantum mechanics!

At this point the reader is invited to pause the video and follow Bell’s argument, if the reader has not already done so, or if the general mathematical formalism is not appreciated the reader should then read the Mermin version of the EPR paradox which spells out the same line of Bell’s gambit in a specific example and therefore a more accessible and less symbolic manner. Or, for those of you who just want to enjoy the game, simply continue reading.

Bell’s Gambit Accepted

Jose Raul Capablanca tells us that the best way to refute a gambit is to accept it.

The traditional approach here is to accept Bell’s gambit, and allow reference to this basic assumption (Equation 1) as the assumption of “local realism”. Unfortunately this line of reasoning has led to what is usually a losing game (a poor or inconsistent understanding of the physics). One line follows the acceptance of the gambit with pursuit of ever-smaller “loopholes” which will enable experiments to remain in accord with Bell’s principle of local realism. Some experimentalists have become quite stubborn in their insistence that all such loopholes are closed , and if this is true than this line of counterattack is truly over. Others maintain some loopholes remain open. Some of the loopholes proposed certainly seem desperate, while others – not so much. Most notably the “detection efficiency loophole” or “fair sampling loophole” appears quite compelling, and we will see that our suggested line in this piece, Bell’s gambit declined, transposes into something which at least seems quite similar to the detection efficiency loophole. But you will see this later.

The only other line of counterattack following acceptance of Bell’s gambit is to pursue a line which is not dependent on any local realism. This desperation leads to a wide variety of chaotic lines, most of which I believe should simply be resigned. Some people suggest that superluminal signalling is possible (even though no evidence exists for superluminal communication, and signalling should enable communication), or that “multiple universes” might be required (on the microscopic level only?) to explain the behavior, and various other hand-waving. Perhaps even stranger is that some pursue the total denial of objective reality and the suggest that the mere conscious registering of a measurement somehow physically changes the world. At this point it appears we have taken a line of self delusion rather than admit that we have made a blunder.

The trouble is not that these ideas have no merit, it is that they contradict the very point of studying physics to begin with. In fact such “spooky” (a word used all too often in these discussions) lines of reasoning can be taken at the macroscopic level as well. There are many things which cannot be named. There are no shortages of mysteries and if this is your pursuit, I encourage you. I also enjoy such pursuit. However the general goal of a communicable physical theory is precisely to describe some subset of our observations of the world in a consistent and useful model. If our efforts in producing such a coherent model wind up with an incoherent model we need to call spooky, it’s time to admit that we have lost the match and try another strategy. Let’s not forget the object of the game now.

Perhaps we should step back and look at places where we may have blundered in our attempts to understand the physics and return again to the board after a short break.

Bell’s Gambit Declined

Usually pop science reporters are not scientists themselves, in that it is their job to report on what scientists have hypothesized and tested rather than to hypothesize and test things themselves. So you might be surprised then to see that Forbes reporter Chad Orzel hits the nail exactly on the head in his article on “quantum loopholes”:

Quantum particles […] are more strongly correlated than possible with any theory in which the measurement outcomes are determined in advance.

OK, so in much of the article he shows that he has been trapped into following the Bell’s Gambit Accepted line, but in this passage he correctly assesses the content of Bell’s so-called “principle of local realism”. The principle does not include all local realistic theories, but only those local realistic theories in which the outcome of a measurement is exactly determined in advance!

This gives us a line of attack which enables us to decline Bell’s gambit, as we know from basic measurement theory, information science, as well as chaos theory, that with a finite ( i.e. limited ) amount of information $\vec \lambda$ it is impossible to predict the results of future measurements to arbitrary precision. Thus we could say that Bell’s principle of local realism isn’t realistic at all.

But that’s not all.

Bell’s gambit also allows for only two outcomes after the electron with state 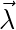$\vec\lambda$ enters the apparatus: either it orients itself aligned upwards to the field gradient and is deflected upward creating a detection event at the upper plate or it orients itself downwards to the field gradient and is deflected downward creating a detection event at the lower plate. This doesn’t allow for the electron to be deflected elsewhere upon entering the device, including internal absorption or reflection, nor does it allow for the electron to arrive at a dead zone on the detector to not register at all. After all, the detector is never going to have 100% efficiency and so the assumption that 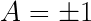$A=\pm1$ cannot be correct. This is where the Bell’s gambit declined line can transpose to look something like the detection efficiency loophole or David Bohm’s “local variable plus nondetection” model. This latter model indeed can explain the predicted (and observed) probabilities while still being a local and realistic theory.

A bifurcation diagram showing a chaotic system which can branch or quantize chaotically.

To summarize: we can deny that Bell’s equation 1 contains all local theories, because it clearly contains only those local theories which include deterministic binary measurement. This refutation opens up an entirely different line of play in our chess game of understanding quantum mechanics.

We can for example describe the result of the Stern-Gerlach experiment to be at first binary (the electron will go one way or the other), but then take the probability of detection to depend on the original angle of the electron spin. This is the simplest line to play following Bell’s gambit declined, as it enables a local theory consistent with predictions of QM with a minimum of added machinery. We can visualize the electron reorienting itself as it experiences torque in the inhomogenous magnetic field, and then losing some of it’s likelihood of detection (via internal state changes or some sort of deflection) in the process.

However other lines are also possible. The initial binary choice could involve external probabilities as well, and the detection probability could have other dependencies.

Variations after Bell’s Gambit Declined

There are some potential refutations to Bell’s Gambit Declined. One such refutation is to assert that the state $\vec \lambda$ in Bell’s description of local realism can contain not just the internal state of the electron but also every possible externality to arbitrary precision. If this is the case, then indeed the experimental result must be a function of this vector. There is no longer any room for probabilistic measurement if every possible external factor is now included. There is no longer any room to decline the gambit. However such a construction leaves much to be desired. Not only is the size of $\vec \lambda$ now necessarily uncountably infinite, but the elements of it are also infinite. The assumption of a “calculable function” now no longer seems to hold. This is an interesting variation but one that appears to be more desperate for the player who is trying to refute Bell’s Gambit Declined. It doesn’t appear to be a comfortable position to play.

Another potential refutation could come from asking further details of the external factors that can affect the measurement and seeking to poke holes in the exact physical model which appears as the game progresses, and the players continue to refine their physical model of the system at hand (electron + inhomogeneous field + detector apparatus). In this case there will be many other battles over details, but at stake will not be whether the system could be classified as local or nonlocal, but over other details, for example of electron structure or the nature of the interaction with the inhomogeneous magnetic field.

Endgame?

So what then is an electron exactly, and what possible interactions take place as an electron moves through an inhomogeneous magnetic field? Well, good questions – and ones that you aren’t going to find all the answers for right here today.

Perhaps however you have found a way to open your exploration of these issues which doesn’t end in a quick checkmate or stalemate. There are plenty of ways that a model electron could behave, locally and realistically, to obey the laws of quantum mechanics. However there are no ways that it could pass through a Stern-Gerlach device such that our measurement is precisely determined in advance by finite internal or hidden variables in the electron. This is what we have really learned from Bell, Aspect, et al.

Thank you and see you all next time.

Acknowledgements

Agadmator’s chess channel.

Thanks to Agadmator for the vocabulary and format of this post 🙂